Individual response

[R_cubed]

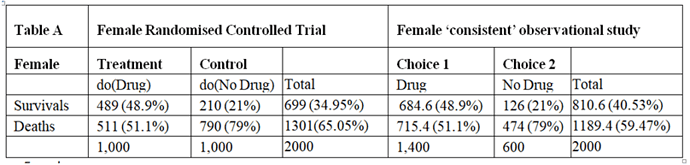
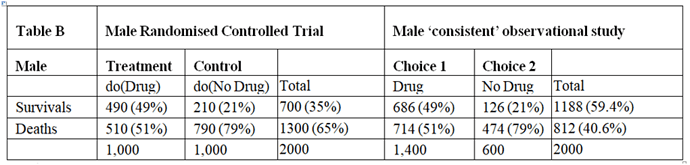
Blockquote
If you are going to claim that my position is “logically false”, then please show me that it relies on a logically invalid syllogism. Alternatively, please stop appropriating terms that have precise definitions in order to borrow authority for your position.

While I think the entire history of human beings learning things long before randomization was discovered is proof enough, Royall already rebutted this demand for randomization in the paper I cited in his discussion of the Likelihood Principle:

Blockquote
Statistical theory explains why the randomization principle is unacceptable. It does this in terms of the concepts of conditionality (ancillarity) and likelihood… The conditionality principle asserts that when there is an ancillary statistic C present, inferences should be based upon the observed value of C. The problem this creates for the randomization principle is that the statistic representing the result of the randomization is ancillary; thus the conditional randomization distribution is degenerate, assigning one to the actual allocation used … the only “inference” on the observed data is “I saw what I saw”.

My in thread post of a quote from Dennis Lindley RE: randomization being useful but not necessary is also relevant.

Individual response

@f2harrell wrote: Blockquote Those that are prospectively planned with a significant amount of resources used to collect high-quality data without much missing data, for which it is still likely that important confounding by indication can ruin the study, For the sake of discussion, I’d like to explore the relative merits of random experiments vs observational research from a decision theoretic viewpoint. Dennis V. Lindley wrote the following on the relevance of randomization that deserves…

Design considerations are very important before the data is collected (for the experimenter), but the Likelihood Principle does not use that to create “hierarchies of evidence” after the data is collected. There should be no a priori reason for a reader to grant randomized studies greater weight than observational ones simply by a pre-data design criterion.

Goutis, C., & Casella, G. (1995). Frequentist Post-Data Inference. International Statistical Review / Revue Internationale de Statistique, 63(3), 325–344. Frequentist Post-Data Inference on JSTOR

Blockquote
The end result of an experiment is an inference, which is typically made after the data have been seen (a post-data inference). Classical frequency theory has evolved around pre-data inferences, those that can be made in the planning stages of an experiment, before data are collected. Such pre-data inferences are often not reasonable as post-data inferences, leaving a frequentist with no inference conditional on the observed data.

@AndersHuitfeldt

(2) If we rely on statistical evidence, to what extent should data from randomized trials be preferred over observational data?

None of this is important if powerful interests can manipulate what studies are published, and which ones are not, via subversion of the peer review process, which Ioannidis discusses in those papers.

Much to the disappointment of those who want easy answers, there are no rules based upon design features alone, that can decide this a priori, especially when there is an appreciable probability of fraud or deception. My position is that needs to be done on a case by case basis, according to formal decision theoretic principles. Michael Rawlins presents many historical examples of medical learning from what we know are imperfect data, that many EBM proponents would find problematic.

De Testimonio: on the evidence for decisions about the use of therapeutic...

The world we have to deal with are:

1. large economically and politically powerful, and coordinated actors who can afford to produce randomized designs (or the illusion of them) vs
2. disorganized and politically weak agents who might be able to rebut biased presentations of RCTs with observational evidence or approximate the relevant RCT when powerful interests have no incentive to conduct a credible RCT.

That is the problem I am worried about, and am working out how to rigorously formalize the notion.

Related Reading

Time to assume that health research is fraudulent until proven otherwise? -...

Rubin, D. B. (1992). Meta-Analysis: Literature Synthesis or Effect-Size Surface Estimation? Journal of Educational Statistics, 17(4), 363–374. https://doi.org/10.3102/10769986017004363

Blockquote
In contrast to these average effect sizes of literature synthesis, I believe that the proper estimand is an effect-size surface, which is a function only of scientifically relevant factors, and which can only be estimated by extrapolating a response surface of observed effect sizes to a region of ideal studies. This effect-size surface perspective is presented and contrasted with the literature synthesis perspective.

In a Robust Bayesian Meta-Analytic approach, design considerations could be treated as a nuisance factor, and integrated out.

There are some errors in statistical reasoning here, but the main points are important.

Evidence-based medicine: can the evidence be trusted?

Blockquote
Seemingly well designed, executed, and reported, RCTs with exciting results can also be misleading due to the hijacked research agenda. These trials are designed to deceive and the methods of deception are alarmingly simple, but effective. The main tactics used relate to the choice of comparators, the choice of outcomes, and the manipulation of statistics to produce desired outcomes, and selectively report them.

Nothing in EBM textbooks or literature prepares a scholar for the adversarial context of the “real world.”

[f2harrell]

A good discussion on individual response/personalized medicine has been going on in twitter with several tweets from Judea Pearl. I hope that we can summarize and refactor that discussion here. My suggestion for doing so is to take a hypothetical but realistic example where we have a treatment benefit for females and an apparent benefit for males based on a randomized clinical trial, but under the surface there are really two populations of males based on the presence or absence of a genetic variant that was not designed to be collected and analyzed in the RCT. Males with the initially hidden variant have a disastrous outcome if treated. I’ll leave it to others to insert some numerical examples for the basis of discussion.

[ESMD]

@f2harrell

hypothetical but realistic example

And therein lies the rub.

Hypothetical example:

A variant of a certain allele, when present on the Y chromosome (i.e., it only has the potential to occur in men), causes a fatal adverse drug reaction when carrier men are exposed to a certain medication. Only a small fraction of all men (e.g., men from a certain limited geographic location in the world) might carry this allele. No men from this geographic location were included in the RCTs used as the basis for drug approval.

Hypothetical AND Realistic example:

None (?) I can’t think of a single recognized clinical scenario that would conform to the hypothetical scenario above. Maybe others can offer one…

[Pavlos_Msaouel]

@ESMD

I can’t think of a single recognized clinical scenario that would conform to the hypothetical scenario above

You are very correct and these twitter conversations are the ones I mentioned above recapitulating old Fisher vs Neyman debates, which contradicts the claim that 20th statistics was supposedly causally blind.

In contemporary terms, Neyman was focused on estimating the average treatment effect (ATE) in a randomized controlled trial (RCT). This can be zero even when some or all patient-level treatment effects differ from zero. Because of this he was interested in the long-run operating characteristics of statistical procedures under both random sampling and random allocation, which we discussed here. This is very unrealistic and that is why we essentially never use it in practice, at least not in medicine.

Fisher on the other hand did not care about the ATE and instead focused on a form of abductive inference from RCTs assuming only random allocation and not random sampling. He accordingly tested the sharp null hypothesis that the effect is zero for every single unit. This assumes it is unrealistic to expect that there is benefit for one subgroup and detriment to another with both equalizing to zero.

@Stephen, an applied statistician with tons of experience and thus closer to the Fisherian practical view, summed this up here as: 'Fisher’s null hypothesis can be described as being, “all treatments are equal”, whereas Neyman’s is, “on average all treatments are equal”.’ The ATE framework can also violate Nelder’s marginality principles, which may be one conceptual source of disagreement behind the Lord’s paradox debates with Pearl.

In the contemporary causal inference world, these considerations are well summarized, e.g., by Guido Imbens and Donald Rubin here.

This disconnect between theory and practice can yield endless debates, which can be quite fruitful and useful to follow albeit exhausting for participants.

[ESMD]

**Addendum after further reflection and modification of the hypothetical scenario described in post 198 above- the modification is designed to illustrate how drug regulators approach rare adverse events:

The reason why I said that I couldn’t conceive of a “realistic” clinical scenario exactly like the hypothetical one above, is that I couldn’t think of an example of an idiosyncratic drug reaction that is known only to occur in males. The scenario becomes much more plausible if we don’t require that the underlying genetic predisposition for the adverse reaction occurs only in males. Instead, let’s imagine that there could be a genetic allele present in a small number of men OR women, that confers risk for a fatal drug hypersensitivity reaction (as described below).

Let’s pretend that the new drug in question is an antimicrobial designed to combat a chronic, hard-to-treat infection. In a clinical trial, its ability to “cure” patients of their infection was compared with the “standard of care” antibiotic. Let’s further pretend that the new antibiotic performed very well in the trial- a much higher proportion of patients treated with the new antibiotic were cured compared with those treated with the standard of care antibiotic. This effect was seen in both men and women, but, unfortunately, one male subject died within 4 days of exposure to the new antibiotic from a multi system hypersensitivity reaction.

What would be the likely outcome from the above trial? Answer- it is very unlikely that the new antibiotic would be approved by the drug regulator. The regulator would be concerned about the potential for additional idiosyncratic reactions to the new drug following marketing. After observing only one event, it would be difficult to identify any occult genetic cause (if present). Therefore, the regulator would realize that prescribers wouldn’t be able to mitigate the risk through pre-treatment patient screening. Furthermore, seeing such an event occur in a modestly-sized trial would raise serious red flags about the potential frequency of this adverse event in the wider target population if the drug were to be approved.

In theory, idiosyncratic reactions are possible with most drugs on the market. But because these types of reactions are, for the most part, known to be very rare, a regulator would become very concerned if such an event were to be captured during a typically-sized clinical trial.

Now let’s modify the scenario, such that the (unknowingly) genetically “vulnerable” male patient had not been invited to participate in the trial. The trial again generates impressive efficacy results, but this time, no cases of hypersensitivity reaction occur. The antibiotic is approved by the regulator. But lo and behold, within a week of its approval (now in the “postmarket” setting), the regulator starts receiving sporadic case reports describing fatal hypersensitivity reactions among patients treated with the new antibiotic. Now what? Answer: This situation will become an urgent priority for the regulator, with letters of warning sent to prescribers, and, perhaps, even instructions to stop prescribing the drug. There will be a concerted effort to try to estimate the frequency of such events.

The more people that have been exposed to the drug before such events are reported, the more confident the regulator can be that the incidence of the idiosyncratic reaction, in target population for the drug, is likely to be “acceptably” low (with the “acceptable” rate defined by the regulator). This is why, even though idiosyncratic reactions have been reported with many drugs, many of them nonetheless remain on the market to this day. If a drug has unique and important benefits for treating a serious condition, and if its risk/benefit profile, for the vast majority of patients who use it, is expected to be favourable, then more harm than good can come, at a population level, from withdrawing the drug than leaving it on the market with cautionary advice to prescribers (e.g., to be on the alert for early signs of hypersensitivity reaction so that the drug can be stopped promptly). In some cases (e.g., certain antiepileptics), we have identified genetic alleles that increase risk (allowing us to screen patients before treatment), but in many cases we have not. This is an accepted risk with the practice of medicine.

[HuwLlewelyn]

I am not sure what Judea Pearl was trying to do by offering this example based on alleles and tasty medication. His and Scott’s paper contains the necessary data to illustrate their argument of how to place bounds on the probabilities of 4 counterfactual situations of (1) survival on control and treatment (2) no survival on control but survival on treatment (‘benefit’), (3) survival on control but not on treatment (‘harm’) and non-survival on control and treatment. I proposed one hypothetical narrative that would make sense of their data from a clinical point of view (see Individual response - #65 by HuwLlewelyn). With imagination, there could be many such appealing narratives but the allele / tasty drug example as proposed by Judea Pearl does not appear to be one of them.

It seems to me that dreaming up appealing illustrative narratives is not the issue. From my viewpoint there are 4 important questions:

1. The paper’s use of the word ‘benefit’ and ‘harm’ in a counterfactual situation differs from that used when describing the probabilities of an outcome conditional on control and treatment and designating the treatment beneficial or harmful.
2. If we could derive probabilities for the above, how would they be used to make medical decisions by also taking into account probabilities of adverse effects and their various utilities (i.e. effects on well-being)? In other words, what is the purpose of calculating probabilities of these 4 counterfactual situations?
3. They are estimating the probabilities of counterfactual situations that are by definition inaccessible for the purposes of verification or calibration, unlike other models of prediction for example.
4. In view of (3) are their assumptions and reasoning about using various results from RCTs and observational studies to arrive at inequality probabilities of these counterfactual situations sound, culminating on page 8 of the paper?

[f2harrell]

@Pavlos_Msaouel

@f2harrell

So I conclude that what I was advocating applies to disease-free survival time but not to overall survival.

Exactly! And this key point becomes clear when we draw the causal graphs. Otherwise it is very hard to see what the modeling challenges are here.

@Pavlos_Msaouel I want to rethink this and disagree with you a bit.

The problem with overall survival (OS) in oncology studies stems solely from the existence of rescue therapy that can prevent or delay death (and can also prevent cancer recurrence but we are talking mainly about post-recurrence changes in therapy). I posit that most any method that tries to estimate OS will be hard to interpret. The only easy clinical interpretation comes from estimating things like the probability of either the need for rescue therapy or death. A state transition model can easily distinguish between need for rescue therapy with and without a later death, and it can count death as worse than rescue therapy. State occupany probabilities can be computed, by treatment, for death, rescue tx or death, rescue tx and alive, etc.

[Pavlos_Msaouel]

Love it! Obviously there are currently differences but this is an open problem and glad to see you using your skills and experience to address it your way.

In fact, if I recall correctly, part of the motivation for writing that DFS vs OS post at the time was that you had posted a comment on another datamethods thread at the time talking about your state transition model approach in the context of COVID-19 trials. Intuitively I can see connections but unable to go deeper, in part also because the cancer that truly motivates my research (renal medullary carcinoma) is highly aggressive and we are not yet at the point therapeutically where we need to use new methods for survival estimation. Thus, I think about this topic far less than I do other challenges.

While our team has very elaborate and efficient Bayesian non-parametric models we use to attack this problem, it is not certain that they will be the optimal approach. A major reason why is that they are time-consuming, hard to intuit/interpret, and lack user-friendly tools. On the other hand, you are an expert with decades of experience at creating popular and powerful modeling tools for the community. Would love to see your group approach these problems.

[f2harrell]

Thanks Pavlos. Ordinal longitudinal Markov state transition models have lots of advantages of interpretation as detailed here, besides being very true to the data generation process. Advantages come from the variety of causal estimands through the use of state occupancy probabilities, and the fact that all of these estimands are simple unconditional (except for conditioning on treatment and baseline covariates) probabilities. What I thin k is needed to make this work in your context are

• rescue therapies must have a clinical consensus around them to be considered for the list (and note that you can distinguish various levels of “rescue” with an ordinal outcome, e.g. surgical vs chemo vs radiation vs chemo+rad)
• in a multi-center RCT the practice patterns for use of rescue therapy are fairly uniform or can be somewhat dictated by a protocol

To me the only problems that are really hard to solve in this context are the existence of non-related follow-up therapies and non-related causes of death such as accidental death.

[Pavlos_Msaouel]

I think these excellent considerations deserve an ongoing discussion/panel particularly with regulators such as the FDA because the challenge is becoming progressively more common across diseases.

[f2harrell]

Right now I’m trying to start just such a project at FDA but for neurodegenerative disease.

Presently at FDA rescue therapy is somethat that is worked around rather than directly addressed, thinking of it as more of a censoring event than an outcome. That always makes results hard to interpret to me.

[HuwLlewelyn]

I have gone over the paper again carefully. The assumption on which the whole paper is based is ‘consistency’. To quote from the paper: “At the individual level, the connection between behaviors in the two studies relies on an assumption known as ‘consistency’ (Pearl, 2009, 2010), asserting that an individual response to treatment depends entirely on biological factors, unaffected by the settings in which treatment is taken. In other words, the outcome of a person choosing the drug would be the same had this person been assigned to the treatment group in an RCT study. Similarly, if we observe someone avoiding the drug, their outcome is the same as if they were in the control group of our RCT. In terms of our notation, consistency implies: P(yt|t) = P(y|t), P(yc|c) = P(y|c).”

However, according to their example data for females:
P(yt|t) = 489/1000 = 0.489, P(y|t)= 378/1000 =0.378, P(yc|c) = 210/1000=0.210, P(y|c)=420/600 = 0.7
So that P(yt|t) ≠ P(y|t), P(yc|c) ≠ P(y|c)

According to their example data for males:
P(yt|t) = 49/1000 = 0.49, P(y|t)= 980/1400 = 0.7, P(yc|c) = 210/100=0.210, P(y|c)=420/600=0.7
So that P(yt|t) ≠ P(y|t), P(yc|c) ≠ P(y|c)

This means that the assumption of consistency is not applicable to the paper’s example data of their RCT and observational study. However, they go on to make the calculations nevertheless “based on this assumption (i.e. of ‘consistency’), and leveraging both experimental and observational data, Tian and Pearl (Tian and Pearl, 2000) derived the following tight bounds on the probability of benefit, as defined in equation (3): P(benefit) = P(yt, y′c). Therefore the estimated probability bounds in their inequality equation (5) do not follow from their assumptions and reasoning. However, by applying these probability bounds they arrive at point estimates of the probability of counterfactual ‘benefit’ and ‘harm’ (the latter are defined in my previous post (Individual response - #205 by HuwLlewelyn).

I created new example data for males and females where the RCT data were identical and the proportions choosing to take the drug and not to take it were the same as in their observational example. However in my new observational study data, P(yt|t) = P(y|t) and P(yc|c) = P(y|c) so that the assumption of ‘consistency’ could be applied. I then applied their calculations to this data that I had created.

Instead of getting point estimates I got probability ranges. For females the probability of ‘benefit’ counterfactually (e.g. by giving treatment, turning the clock back and giving placebo) was between 0.279 and 0.489 and the probability of ‘harm’ was between 0 and 0.21. For males the probability of ‘benefit’ was between 0.28 and 0.49 and the probability of ‘harm’ was between 0 and 0.21. (p(Harm) = p(Benefit) – CATE, which was 0.279 and 0.28 for females and males respectively). The calculations are in the Appendix below. If the assumption of consistency is valid, we can tell all this from the RCT alone: p(Benefit) ≥ Pr(yt) - Pr(yc) and P(Benefit) ≤ Pr(yt) and p(Harm) = p(Benefit) - (Pr(yt) - Pr(yc)), so that the observational study adds nothing in this context. As we have discussed already, observational studies can be useful in other ways such as detecting adverse effects.

Appendix
Female RCT and ‘consistent’ observational study

Calculations for females (replacing those on pages 8 and 9 in the paper), when Pr indicates that the probability is from the RCT and Po indicates that the probability is derived form the observation study:
P(Benefit) ≥ 0
P(Benefit) ≥ Pr(yt) - Pr(yc) = 489/1000 -210/1000 = 279/1000 = CATE = 0.279
P(Benefit) ≥ Po(y) - Pr(yc) = 0.4053 – 0.21 = 0.1953
P(Benefit) ≥ Pr(yt) - Po(y) = 0.489-0.4053 = 0.0807

P(Benefit) ≤ Pr(yt) = 489/1000 = 0.489
P(Benefit) ≤ Pr(y’c) = 790/1000 = 0.79
P(Benefit) ≤ Po(t, y) + Po(c, y’) = 686/2000 + 474/2000 = 0.343 +0.237 = 0.58
P(Benefit) ≤ Pr(yt) − Pr(yc) + Po(t, y′) + Po(c, y) = 0.489-0.21 +0.357+0.063 = 0.6997
Therefore:
0.279 ≤ p(Benefit) ≤ 0.489 and (0.279-0.279) = 0 ≤ p(Harm) ≤ 0.21 = (0.489-0.279)

Male RCT and ‘consistent’ observational study

Calculations for Males (replacing those on pages 8 and 9 in the paper):
P(Benefit) ≥ 0
P(Benefit) ≥ Pr(yt) - Pr(yc) = 490/1000 -210/1000 = 280/1000 = 0.28 = CATE = 0.28
P(Benefit) ≥ Po(y) - Pr(yc) = 0.406 – 0.21 = 0.196
P(Benefit) ≥ Pr(yt) - Po(y) = 0.49-0.406 = 0.084

P(Benefit) ≤ Pr(yt) = 49/1000 = 0.49
P(Benefit) ≤ Pr(y’c) = 790/1000 = 0.79
P(Benefit) ≤ Po(t, y) + Po(c, y’) = 686/2000 + 474/2000 = 0.343+0.237 = 0.58
P(Benefit) ≤ Pr(yt) − Pr(yc) + Po(t, y′) + Po(c, y) = 0.49-0.21 +0.357+0.63 = 0.7

Therefore:
0.28 ≤ p(Benefit) ≤ 0.49 and (0.28-0.28) = 0 ≤ p(Harm) ≤ 0.21 = (0.49-0.28)

[s_doi]

I agree. To use the words of Gelman, I think it’s helpful to develop statistical methods in the context of applications, and also to work toward theoretical understanding, as Pearl has been doing. However, the push towards theoretical understanding from Pearl has been around for a long time yet it lacks any concrete practical application (except for the theoretical ones like in this thread). No clinician in this thread so far has endorsed any of this as helpful for clinical decision making so I wonder where we are heading? It would be good if someone on this thread could post a real world example of where a problem has been solved using the theoretical explanations posted in this thread.

[HuwLlewelyn]

Thank you @s_doi. There seem to be many reasons for the failure to implement these theoretical ideas. One is difficulties in communication. For example, ‘benefit’ and ‘harm’ as an individual response in the context of counterfactual situations has a completely different meaning to benefit and harm arising from the use of an intervention. This is illustrated in the paper’s conclusion that in females the probability of individual ‘harm’ from treatment is zero when more people die on the treatment than on placebo. The latter describes the response of groups of individuals and is subject to stochastic variation, which as @Stephen pointed out, prevents estimation of individual response. The probabilities of outcomes can be substantiated by experiment whereas we cannot in reality turn the clock back and create a counterfactual situation to substantiate individual response.

We have a rationale for making decisions based on outcome probabilities but it not clear how probabilities of ‘individual benefit’ or ‘individual harm’ would change these decisions. From my calculations, it does not change the information available to us from RCTs at all as p(Benefit) ≥ Pr(yt) - Pr(yc) and P(Benefit) ≤ Pr(yt) and p(Harm) = p(Benefit) - (Pr(yt) - Pr(yc)). What we need is better predictive information (e.g. when everyone dies by using parachute with a big hole in the canopy but no one dies with a proper parachute). In this situation an observational study would be as good as an RCT but reason alone as good as both, making the studies unethical! However, the reasoning must be sound, which includes checking that the assumptions about the data are consistent with the data (or at least not clearly inconsistent).

[s_doi]

Thanks @HuwLlewelyn , I think your summary brings great clarity to this discussion and makes a lot of sense. It also reminds me of a quote in some other thread attributed to Vineet Tiruvadi that seems to apply to the framework in this thread “if you start with the wrong framework then the ability to do complex analyses may seem like it’s giving insight, but what you’re mostly doing is studying how wrong your framework is”

[f2harrell]

This discussion is incredibly helpful. @HuwLlewelyn joins @Stephen Senn in being the most impressive scientists I’ve known in their abilities to cut through arguments of others and to make cogent new arguments. It confirms what @Stephen has argued repeatedly that principles of experimental and clinical trial design must be brought to causal inference about treatment effects. The discussion also confirms my previous feeling that outside of special situations (such as analysis of treatment effects within RCTs compensating for non-adherence to treatment) causal inference remains a theoretical nicety and a great thought organizer but has not yet been translated to practical application in treatment evaluation. Hence the lack of uptake on the challenge put at https://discourse.datamethods.org/t/examples-of-solid-causal-inferences-from-purely-observational-data.

[HuwLlewelyn]

One area where causal inference might be translated to a practical application in treatment evaluation is when taking HTE into consideration. This was a tweet that I addressed to Judea Pearl recently to which he did not reply:

In RCTs Irbesartan reduces risk of nephropathy. HbA1c & AER are risk factors. According to ‘causal’ medical theory, Irbesartan should reduce AER but not HbA1c. For HTE, should risk reduction be estimated due to that of AER alone & not HbA1c? How does CI notation express this?

How would @Stephen and others in this discussion design a study to answer this question?

[Stephen]

I have yet to study Huw’s reply in detail but on a brief read I think that it gets to the nub of the argument. It seems baffling to me that consistency is considered to be reasonable or practical. However, I wonder if in fact M&P depends on more than just “that an individual response to treatment depends entirely on biological factors, unaffected by the settings in which treatment is taken”. The individuals contributing information from the observational studies are not the same individuals as in the RCTs. Thus we have to be able to assume that the two sets of individual are exchangeable to the extent needed in order to be able to solve for the unknowns. I do not consider this to be a reasonable assumption and referred to “study effects” as being a problem. The TARGET study is an excellent example of the problem Lessons from TGN1412 and TARGET: implications for observational studies and meta‐analysis - Senn - 2008 - Pharmaceutical Statistics - Wiley Online Library
The way that study effects are dealt with in conventional statistical approaches is either by declaring them as fixed and hence eliminating them by contrasts or as declaring them as random and then trying to estimate the variance component. All of this was extensively developed in connection with incomplete block designs by the Rothamsted school in the period 1925-1945.
My view is that adding observational data does not pull the rabbit out of the hat. Adding extra equations does not necessarily render a system identifiable, in particular, if in doing so one adds more unknowns.

[HuwLlewelyn]

I would like to sum up following @Stephen’s and my latest skirmish with Judea Pearl on Twitter. He wrote that I was wrong to assume that p(Yt) from the RCT should have been equal to p(y|t) from the observation study. However he reasserted that p(y|t) was equal to p(yt|t), the latter being the result of a ‘Level 3’ or imaginary RCT result that applies to choosers (it can be imagined after reasoning from other established beliefs but cannot be done in realty). It seems that the assumption of ‘consistency’ is therefore a Level 3 or imagined result of p(yt|t) that is equal to (y|t) the observation study result. This assumption of ‘consistency’ is therefore unverifiable and un-refutable by study and based on personal belief leading to a forceful assertion.

The only probabilities supported by reliable data are the results of the RCT. If we are only prepared to rely on the RCT results (but not rely on forceful assertions based on imagination) then all we can conclude is that from counterfactual concepts, p(Individual Benefit) ≥ Pr(yt) - Pr(yc) and P(Individual Benefit) ≤ Pr(yt) and p(Individual Harm) = p(Individual Benefit) - (Pr(yt) - Pr(yc)) as I explained in a previous post. However, the latter probabilities of imaginary individual counterfactual outcomes do not seem to make any difference to practical decisions, which result in the reasoning set out in @Stephen’s Twitter response [See https://twitter.com/stephensenn/status/1617807975858704385?s=20&t=8Kkuwt3CM9K7ceCUYGnSXA].

[f2harrell]

Huw, I and others greatly appreciate your diligence on this incredibly important topic. I tried my very best to get Judea to join us here so that he could try to expand his arguments and provide details as you have done, and also to carefully read all the posts here, but to no avail. But your posts, like those of @Stephen are also highly useful for citing in tweets. If you haven’t done this already, clicking on the 3 dots at the bottom of the post pulls up a chain link symbol that can be clicked on to get the URL that leads directly to a specific reply, for inclusion in a tweet.